Hyper-cores promote localization and efficient seeding in higher-order processes

Abstract

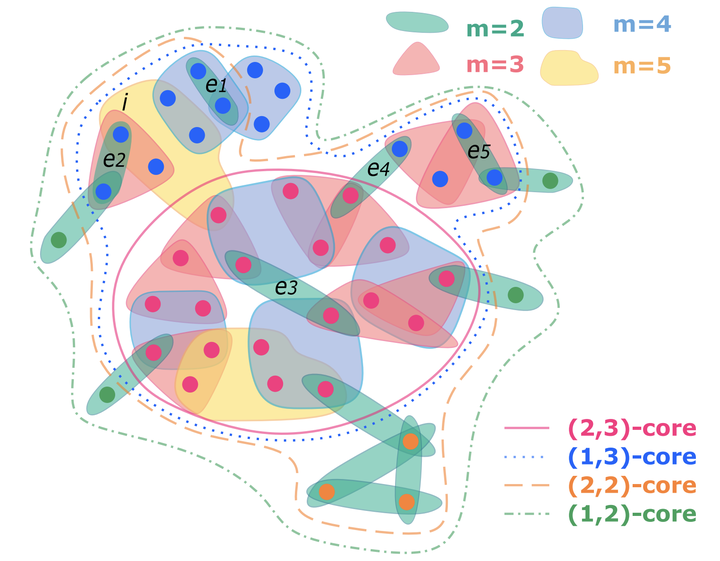
Going beyond networks, to include higher-order interactions of arbitrary sizes, is a major step to better describe complex systems. In the resulting hypergraph representation, tools to identify structures and central nodes are scarce. We consider the decomposition of a hypergraph in hyper-cores, subsets of nodes connected by at least a certain number of hyperedges of at least a certain size. We show that this provides a fingerprint for data described by hypergraphs and suggests a novel notion of centrality, the hypercoreness. We assess the role of hyper-cores and nodes with large hypercoreness in higher-order dynamical processes: such nodes have large spreading power and spreading processes are localized in central hyper-cores. Additionally, in the emergence of social conventions very few committed individuals with high hypercoreness can rapidly overturn a majority convention. Our work opens multiple research avenues, from comparing empirical data to model validation and study of temporally varying hypergraphs.